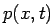 , which
evolves according to the following equation
, which
evolves according to the following equation
Fleming-Viot Processes |
| O. Cybulski, V. Babin, R. Holyst |
We consider the system of the Brownian particles confined in a box
whose boundaries act as a sink of the particles. The annihilation rate
at the boundaries is matched by the branching (birth) rate in the bulk,
thus the total number of the particles is preserved. The process can
be described in terms of the probability distribution function
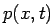 , which
evolves according to the following equation
, which
evolves according to the following equation

and vanishes at the container boundaries. The first term in the right
hand side describes diffusion of the particles, and the second one represents
the branching process. The constant
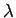 can
be viewed as the Lagrange multiplier controlling normalization of
can
be viewed as the Lagrange multiplier controlling normalization of
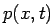 :
:
 .
.
It turns out that the dynamics of the process can be conveniently interpreted in terms of the Renyi entropy
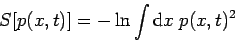
and the Renyi entropy production
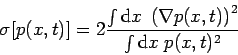
Namely, the Renyi entropy production is monotonically decreasing function of time irrespective of the initial conditions. In the steady state it is equal to the lowest eigenvalue of the Dirichlet Laplacian. More details can be found in our paper.
All the movies below have been encoded with MEncoder. To watch the movies, the DivX codec must be installed on your system.
|
|
|
Shown is the time evolution of the probability distribution function
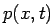 for two different
container geometries. The green-orange colormap is used to visualize the
value of
for two different
container geometries. The green-orange colormap is used to visualize the
value of 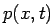 . In the
top pannel of each movie the Fleming-Viot process is presented, while in
the bottom one, regular diffusion evolving from the same initial
configuration is shown.
. In the
top pannel of each movie the Fleming-Viot process is presented, while in
the bottom one, regular diffusion evolving from the same initial
configuration is shown.
|
|
The last movie concerns one possible generalization of the process described above, in which many types (colors) of the Brownian particles are allowed. When particles of different colors collide, both annihilate instantiously. At the same time, randomly chosen particles of the corresponding colors are branched. This way the total number of the particles of each color is preserved.